
On the computational complexity of the Jones and Tutte polynomials. Transversal matroids and related structures. 4 in Mathematical Carnival: A New Round-Up of Tantalizers and Puzzles from Scientific American. Plates 3-4 in Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume. "Computer Recreations: A Program for Rotating Hypercubes Induces Four-Dimensional Dementia." Sci. Mathematische Spielereien (Mathematical Games), Union Deutsche, 1918, 140 pages.ĭewdney, A. Springer-Verlag, New York, second edition, 1996.Ĭzepa, A. Plane answers to complex questions: the theory of linear models. Revisiting the accurate calculation of block-sample covariances using gauss quadrature. The authors demonstrate the startling nature of the fourth dimension.Īssiduously following the schematic, folding begins.Ī hypercube in its properly-folded end-state.īorn, M. The unfolded Hypercube (and the authors pictured, right): Initial construction of the unfolded Hypercube. Sturdy materials are gathered and assembled. However, folding is no more complicated than bringing end to proper end, as indicated in the following schematic. It follows that, to successfully fold a Hypercube, we should adopt the selfsame Method beginning with it unfolded into three dimensions, as shown diagrammatically below. Observe:īelow we have a three dimensional cube unfolded into two dimensional space, a procedure easily completed by a young schoolchild.
#Unfolded hypercube full#
We know full well that the great majority of scientific men will not believe that such results can be practically and immediately realized, but this tract shall prove otherwise indeed provide photographical evidence of the Hypercube’s properly-folded end-state.Īfter considerable reflection, we determined that the most sure-footed Method available to us was the Method of Folding, first pioneered, though never executed, by R.

It seems obvious that if we, in the manner Euclid or Descartes, were to advance only by rigorous Method, placing aside all other commonplaces and never once straying from direct implication, we cannot help but to achieve our intended result. What, then, is to save us from such dissimilation? How are we to guarantee for ourselves the success that other great geometers have won so definitively and demonstrably throughout the ages? The answer is, of course, by recourse to Method. I beg our audience to consider what would prompt such great men of distinction to dismiss Hypercubes as an outright impossibility? Again the senses. Many of our contemporaries have failed in this regard. Once the thinking man is freed of his positivistic shackles, and no longer led astray by the seduction of language and the coarseness of his senses, only then is he prepared to rightly conceive of the fourth dimension. It is our task then, here and elsewhere, to place Natural Philosophy back on the secure path of rational speculation and self-evident ideas.
#Unfolded hypercube free#
That is because Natural Philosophy, so long mired in the muck of empiricism, has made little to no progress in the last three to four centuries since the publication of Boyle’s decadent A Free Enquiry into the Vulgarly Received Notion of Nature. Notwithstanding, we regret to say that no progress has been made, among the community of scientific men, in producing a real Hypercube. Much ink has been spilled and much time spent, on the consideration of Hypercubes. In this exercise, we shall limit ourselves to but four dimensions, only later to generalize our Method to the fifth, sixth, and even seventh dimensions. With our mathematical definitions fixed and clearly explicated, we may, at last, get on with the actual construction of our Hypercube. The implications have been left to the reader as an exercise. The number of nodes in the n-Hypercube is therefore 2 to the n, the number of edges is, the number of squares is, the number of cubes is, et cetera. It may also interest the reader to know, that the number of k-cubes contained in an n-cube can be easily found from the coefficients of, namely, where is a binomial coefficient. Moreover, it is denoted and has Schläfli symbol. Simply stated, a Hypercube is an n-dimensional regular polytope with mutually perpendicular sides. The foremost among them being, What precisely is a Hypercube?
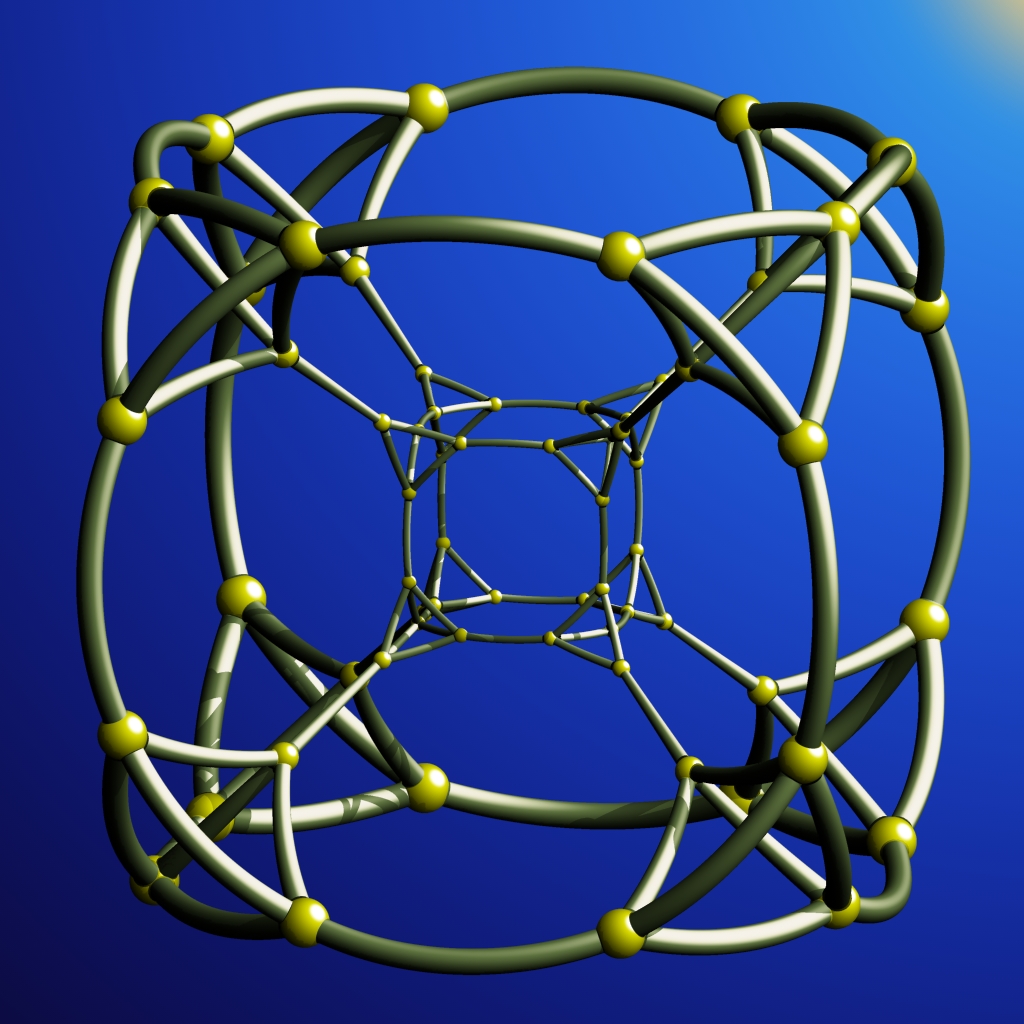
In endeavoring to fold a true 4-dimensional hypercube, we must first, as might be expected, concern ourselves with questions of mathematical definition.


 0 kommentar(er)
0 kommentar(er)
